F.E.D. Dialectics: Dialectical Calculation: Two Theorems

Monday, September 12, 2011

Dialectical Calculation: Two Theorems

Dear Reader,

FYI: Two key generalizations can be constructed upon patterns evident in the "dialectical, purely-qualitative calculations" of my immediately-previous blog-entry here, which was entitled "How to Calculate Dialectically [and purely-qualitatively] --

1.) First generalization / theorem:

|-|-|k+1   =

|-|-|k[ |-|-|k ]   =

|-|-|k "of" |-|-|k   =

|-|-|k "times" |-|-|k   =

|-|-|k x |-|-|k   =

|-|-|k "squared"   =

|-|-|k^2   =

"the <<aufheben>>, determinate, QUALITATIUVELY DIFFERENT self-negation of" |-|-|k    =

~|-|-|k.

This chain of equations, which constitutes the F.E.D. "generic meta-evolution equations", or "generic ontological revolution equations", defines the |-|-|k as "self-reflexive functions", that is, as "subject-[verb-]object identical" operators, expressed "ideo-gram-ically", as a "dialectical-algebraic meta-model", rather than in the form of a "phono-gram-ically"-expressed, phonetic sentence(s) "meta-model".

Indeed, the F.E.D. "Dyadic Seldon Function" for the generic dialectic --

|-|-|k    = |-|-|0^(2^k)     = [ q/1 ]^(2^k)

-- is the general solution to the "meta-finite difference equation(s)" stated above.

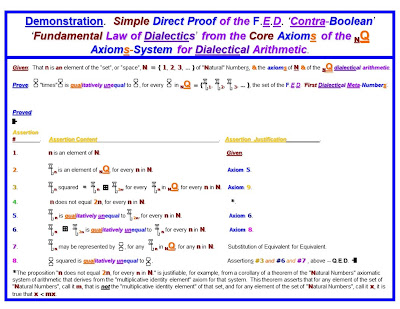
2.) Second generalization / theorem: The Whole-number exponent, or power, of the <<arche'>> equals the number of ontological categories summed [non-amalgamatively] in the expansion of that power-expression.

The <<arche'>>, or originating, initiating, ever-present-origin ontological category of a dialectical categorial progression, is generically represented by the purely-qualitative "meta-numeral" q/1 in the NQ "First Dialectical Arithmetic", whose "meta-number" set is --

NQ   =   { q/1, q/2, q/3, q/4, . . . }, given --

N    =   { 1, 2, 3, 4, . . . }

-- as the number-set of the "Natural" Numbers.

The number-set known as the "Whole numbers" is --

W = { 0, 1, 2, 3, 4, . . . }.

Thus --

|-|-|0^(2^0)   = [ q/1 ]^(2^0)   = [ q/1 ]^1   = q/1;

|-|-|0^(2^1)   = [ q/1 ]^(2^1)   = [ q/1 ]^2   = q/1 + q/2;

|-|-|0^(2^2)   = [ q/1 ]^(2^2) ~= [ q/1 ]^4   = q/1 + q/2 + q/3 + q/4;

|-|-|0^(2^3)   = [ q/1 ]^(2^3) ~= [ q/1 ]^8 =

q/1 + q/2 + q/3 + q/4 + q/5 + q/6 + q/7 + q/8,

etc.

The two statements above can, of course, be proven deductively, given the full axioms-set of the NQ, or of the WQ, dialectical, "purely"-qualitative arithmetic.

For more information regarding these Seldonian insights, please see --

www.dialectics.info

For partially pictographical, ‘poster-ized’ visualizations of many of these Seldonian insights -- specimens of ‘dialectical art’ – as well as illustrated books by the F.E.D. Press, see --