A '''Mind-Tool''' for Scientific Idealization, Self-Clarification, and Discovery.

Dear Reader,

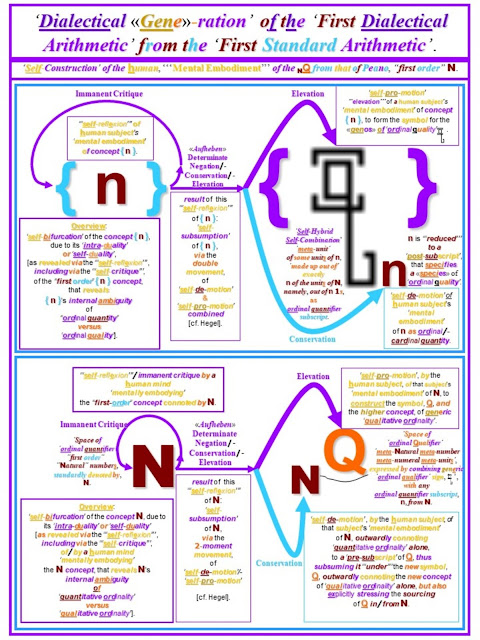
The second axioms-system in the Seldonian ‘‘‘meta-systematic’’’ method of presentation of the progression of the axioms-systems of the Seldonian arithmetics / algebras of dialectics, named _NQ_, is the simplest, most abstract, and least determinate -- in its expressive, descriptive capabilities -- of all of the explicitly dialectical axioms-systems of arithmetic / algebra in that systems-progression.

This does not mean, however, that the _NQ_ system is the least useful system in that progression.

It does not mean that one of the more advanced dialectical-ideographic languages in that languages-progression is always, in every case, to be preferred as the language in which to formulate problems and in which to discover their solutions.

On the contrary, the _NQ_ system is perhaps the most generally / universally useful of all of the systems in that systems-progression -- and the most likely best place to start, for ‘heuristic-algorithmic’ support -- in the formulation of problems and the search for their solutions.

¿Why?

¡Precisely because of its very simplicity!

That abstractness and simplicity gives _NQ_ its methodological power, its power as a heuristic tool, because that abstractness and simplicity facilitates scientific idealization.

Most of the details, complications, and potential distractions that threaten to derail a more -- and a “too soon” -- determinate [but therefore also more “chaotic”, in the sense of Marx] description of a problem, and not even expressible in the language of the _NQ_.

Descriptions of an “external environment” of the central ‘‘‘eventities’’’ [beginning, of course, with the ‘arché eventity’] to be dialectically modeled, and of that environment’s interactions with / actions upon, those ‘‘‘eventities’’’; descriptions of the physical spatiality of those ‘‘‘eventities’’’, and even of their detailed temporality -- all of these details must, at first, be stripped away, in an _NQ_ formulation of a problem, or of a presentation.

All that remains, after that “stripping away”, is a single, kind-of-being ‘arché category’, and the ‘meta-genealogy’ that proceeds out of it through the advancement of an abstracted -- minimal -- form of temporality:  ‘temporal ordinality’.

Regards,

Miguel