Part 09: Seldon’s Axioms Series. Re-Presentation of the nQ Axioms-System as a Whole.

 

 

 

Part 09: Seldon’s Axioms Series.

 

 

Re-Presentation of the _NQ Axioms-System as a Whole.

 

 

 

 

 

 

 

Dear Reader,

 

 

 

It is my pleasure, and my honor, as an elected member of the Foundation Encyclopedia Dialectica [F.E.D.] General Council, and as a voting member of F.E.D., to share, with you, from time to time, as they are approved for public release by the F.E.D. General Council, Encyclopedia Dialectica definitions of the key elements of Seldonian Theory.

 

The 9th text in this new such series is posted both above and below [Some E.D. standard edits have been applied, in the version presented below, by the editors of the F.E.D. Special Council for the Encyclopedia, to the direct transcript of our co-founder’s discourse].

 

 

 

Seldon –

 

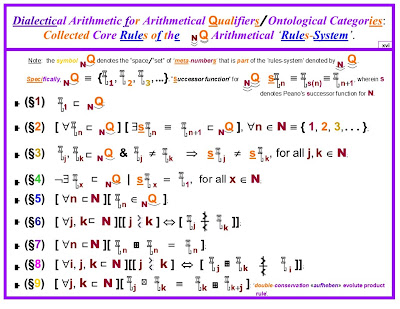
“In our presentations of the core axioms of the _NQ arithmetic for modeling dialectics, we should emphasize the character of that arithmetic as a “non-standard model of the Peano Natural Numbers” –    

“…The [E.D. – by Gödel] incompleteness theorems hold also for higher-order formalizations of number theory.  If only first-order formalizations are considered, then the [E.D. – also by Gödel] completeness theorem applies as well, and together they yield not a contradiction, but an interesting conclusion: Any sentence of [E.D. – “Natural”] arithmetic that is undecidable must be true in some models of Peano’s axioms (lest it be formally refutable) and false in others (lest it be formally provable).  In particular, there must be models of first-order Peano arithmetic whose elements do not “behave” the same as the [E.D. – “standard”] “natural numbers”.  Such nonstandard models were unforeseen and unintended, but they cannot be ignored, for their existence implies that no first-order axiomatization of number theory can be adequate to the task of deriving as theorems exactly those statements that are true of the [E.D. – “standard”] natural numbers.  [E.D. – emphases as in original].”

[J. W. Dawson, Jr., Logical Dilemmas: The Life and Work of Kurt Gödel, A. K. Peters, Ltd., 1997, pp. 67-68.].”

 

“To do so, it would be helpful to organize our nine core axioms in the following new format:

 

Section 1: ‘Peanic Axioms’ [axioms §1–§4; a non-standard version of the first 4, first-order-logic “Peano Postulates”];

Section 2: ‘Bridging Axiom’ [axiom §5; links _NQ to N]

Section 3: ‘Non-Peanic Non-Standard Axioms’ [axioms §6–§9; the axioms which define the major ‘ideo-ontological’ differences distinguishing _N  Q   from N   ].”

 

 

 

 

 

 

 

For more information regarding these Seldonian insights, please see --

 

www.dialectics.info

 

 

 

 

 

 

 

 

 

 

 

For partially pictographical, ‘poster-ized’ visualizations of many of these Seldonian insights -- specimens of ‘dialectical art’ – as well as dialectically-illustrated books published by the F.E.D. Press, see –

 

https://www.etsy.com/shop/DialecticsMATH

 

 

 

 

 

 

 

 

 

 

 

¡ENJOY!

 

 

 

 

 

 

 

 

Regards,

 

 

Miguel Detonacciones,

 

Voting Member, Foundation Encyclopedia Dialectica [F.E.D.];

Elected Member, F.E.D. General Council;

Participant, F.E.D. Special Council for Public Liaison;

Officer, F.E.D. Office of Public Liaison.

 

 

 

 

 

 

YOU are invited to post your comments on this blog-entry below!